What do we know about planetary rings? Quite a lot, actually!

Short version of this blog entry: for everything you ever wanted to know about planetary ring systems, read a new review article by Matt Tiscareno titled, laconically, "Planetary Rings," now available on arXiv. (Thanks to Luke Dones for the pointer.)

And now for the longer version. I most commonly write about papers from Science and Nature for the perhaps not very good reason that those papers are short. Their brevity means that it's pretty easy to wrap my head around an article and write a meaningful post about it in the space of a few hours. Sometimes I write about scientific papers published in Icarus or the Journal of Geophysical Research. These papers are invariably more nuanced and based on a much longer span of research work (so are far more likely to stand up to scrutiny than anything published in Science or Nature), but they also take more time for me to understand and synthesize, usually half a day to a day.

And then there are review papers. By their very nature, it's impossible to summarize review papers, because they are themselves summaries of decades -- or even centuries -- of work by numerous scientists. So I never write about them. But I'm moved to write about Tiscareno's new paper because it contains answers to so many questions I've attempted to research on the Web while writing stories, and failed to find useful resources. In just the first few pages I've learned a lot of things that made me go "hmm" or "aha!"

Early in the paper there's stuff about Roche limits. The Roche limit, as Tiscareno defines it, "is the distance from a planet within which its tides can pull apart a compact object....However, the Roche limit does not actually have a single value, but depends particularly on the density and internal material strength of the moon that may or may not get pulled apart."

This much I knew, but in the following paragraphs he discusses Saturn's small ringmoons as little probes of the ring's physical properties. Ringmoons are chunks of solid material that are resistant to being torn apart by tidal forces. Over time they accumulate a surface dusting of very fluffy material, but that stops when the density of the whole thing (solid core plus fluffy coating) reaches the Roche critical density. The unusual persistence and expanse of Saturn's rings results from the fact that their Roche critical density is lower than for any other planet, approaching a density only 40% that of water ice. This, in turn, results from the fact that Saturn's rings have a much higher proportion of ice to rock than any other planet's, which lowers their overall density. (Tiscareno doesn't get into why Saturn's rings are more icy than rocky; a recent paper by Robin Canup proposes that Saturn ate the core of a Titan-sized moon and left its icy mantle in orbit.)

There's some fascinating discussion of the other giant planets' Roche limits and what they imply about the densities of their moons. For instance, if you smashed up Uranus' "Portia group" of moons (Cordelia, Ophelia, Bianca, Cressida, Desdemona, Juliet, Portia, Rosalind, Cupid, Belinda, Perdita, and Puck) and spread their mass over the area of your orbits, you'd get something with roughly the same density as Saturn's A ring. So why are they moons instead of an A ring? Likely because they're denser (rockier) than Saturn's A ring: "This moon system may be very similar in origin to the known ring systems, except that the natural density of accreted objects is larger than the Roche critical density...so that any moon that gets disrupted by a collision (which ought to have happened many times over the age of the solar system) will simply re-accrete."

Planetary rings are often described as being small versions of the kinds of disks from which planets form. The parallel is useful but it's interesting that the two systems, both of which consist of rotating disks of particles orbiting a large central mass, are flat disks for entirely different reasons. Ultimately, planetary ring systems are flat because of the oblate (equatorially bulging) shapes of planets, which creates an asymmetric gravity field around the planets. Stellar debris disks don't have these asymmetric gravity fields. They are flat, ultimately, because of the large angular momentum of the disk itself. While the two systems have different causes, they both wind up with particles orbiting in a preferred plane because collisions among particles damp out any motion perpendicular to that plane. Saturn, uniquely, has one set of rings aligned with the planet's rotation plane (due to Saturn's equatorial bulge) and a much larger, more distant ring aligned with its orbital plane (the Phoebe ring, which is too far from Saturn for the equatorial bulge to have an important contribution to the orbital evolution of its particles).

The paper reviews the reasoning for why there should be rings at Mars and Pluto and why they have been difficult to detect (if they exist at all). It reviews the evidence for rings at Saturn's moon Rhea and why they likely do not exist. It reviews the detectability of exoplanetary rings.

After considering all these cases of known or theorized actual rings, the paper goes on to a more theoretical treatment of the types of rings and the phenomena found within them. It explains the difference between spiral density waves (which are compressional and propagate outward) and spiral bending waves (which are transverse and propagate inward) and why they're cool: "Spiral waves, especially weak ones, are useful structures that can be thought of as in situ scientific instruments placed in the rings." You can get masses of small moons from spiral density waves, and deduce properties of the rings themselves from bending waves, under the right lighting conditions, near the equinoxes.

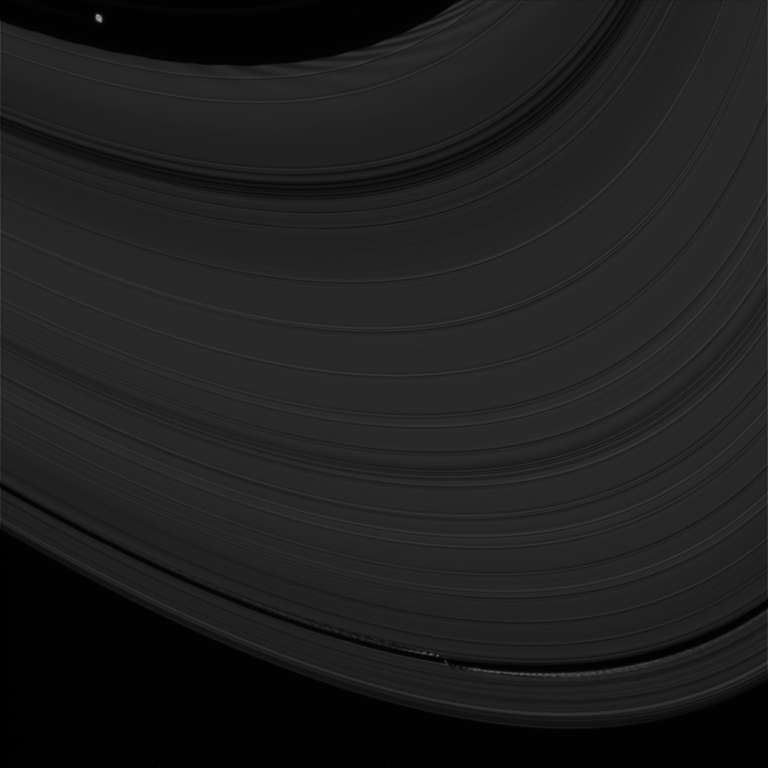
There's a fair amount of mathematics in the article, which I instinctively skip while reading. But when I force myself to go back and read the equations I find they're of a general form that even someone who hasn't written down an equation for 12 years can read. We learn about Keplerian shear, and how the scalloped edges of the Encke gap combined with relatively simple mathematics enabled Mark Showalter and his coworkers to predict the location of, and then discover, the embedded moon Pan in archived Voyager images. We learn about propellers and spokes.

There's more, but like I said, it's folly to attempt to summarize a paper that is itself a summary of the results of four centuries of scientific research. Have you ever looked at Cassini images and tried to figure out what makes those cool structures in Saturn's rings? Have you ever wondered what the difference is between a bending wave and a density wave and a ringmoon wake? Have you ever asked questions like "why doesn't Mars have rings" or "do extrasolar planets have rings?" or "what are they talking about when they discuss 'propellers' in Saturn's rings?" Give this paper a try! You'll also learn about "Propeller Belts" and "frog resonances" and the fact that "The F ring is the granddaddy of narrow dusty ringlets" and it contains "an unseen belt of kilometer-sized moonlets."